When Hips Collide


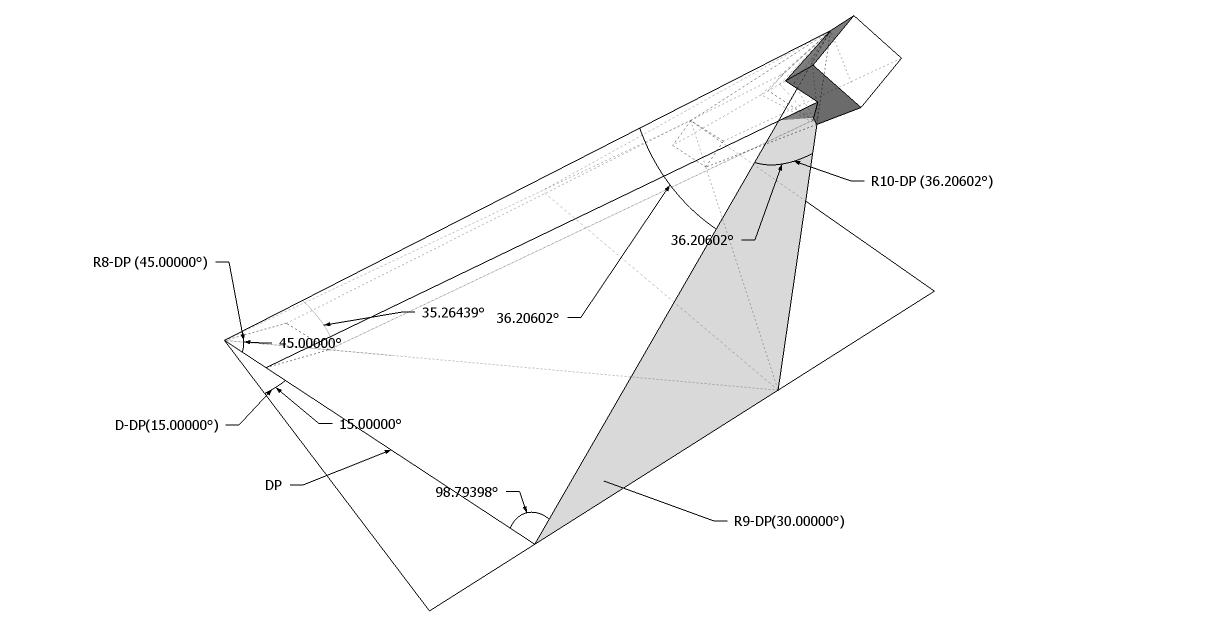
While developing the Tréteaux Angles Trigonometry Formulas Joe Bartok and I have looked at Devers De Pas -- DP, trait carré -- TC and  tréteau à devers from several viewpoints. Now Joe is taking the next step, in developing the claw angles on the hip rafters when they collide. The main concept in Devers De Pas is to develop the footprint of the hip rafter or purlin rafter and use the DP line of the footprint to determine the rest of the points in the drawing that define the miter and claw lines of the intersection of the rafters.

Here's some of the email conversations and drawings from Joe.

____________________________________________________________________________

Spring is just around the corner, I’ll be spending more time outdoors soon and I don’t know if I’ll have this figured out by then, but until then I'm going to keep working on this ...

Sim – I was surfing the ‘net a couple of days ago, researching Devers De Pas and French Drawing, and ended up at your post and Chris Hall’s “X marks the Spot”. Which inspired the following experiment ...

I've made a few changes to the dimensions, the ridges in my model intersect whereas in Chris' model they don't, and I'm only doing a partial joint similar to a claw. Other than a first look and a few notes of his 3D images to understand the basics, I haven't gone back for a peek to see how this is really done.


I've just started the angles on the underside and the look like they're going to be even tougher nuts to crack. Rather than this brute force drawing approach, it might have been more productive to find the tetrahedra, I know they’re hidden in there somewhere.

The intersecting_hips_first_principles_calc.jpg solves for the slopes of the upper faces of the Hips with respect to their traces in plan. The corner angle is known, so we can find the theoretical C5 angles.

These same C5 angles. viewed with respect to the 57.47204° angle between the Hip ridge lines on the roof surface, can be treated as Common Slope Angles, with the 57.47204° angle being W. If we run these figures through a roof framing calculator, the 90° – P2 angles should return 22.59890° and 37.65287°. I can run a calculation like this with a standard Hip-Valley roof and the answers make perfect sense, but in this case, they do not.

The intersecting_hips_compound_angle_calc.jpg is a compound angle solution. At least, it makes sense for the upper surface angles. The bottom surface is actually anyone’s guess, as I’m assuming the 37.65287° behaves the same as in a claw rafter. Given what’s been happening so far, this is unlikely ...

Those Hip rafter footprints are the key to the upper surface angles, because extending the footprint lines define the traces of the upper surfaces in plan. The intersections of the traces determine the endpoints of the claw triangles.


For a first attempt I chose "easy" Hips of square section with intersecting ridge lines. In hindsight, the Hips could have been rotated, rectangular, a 60°-120° parallelogram, and the upper surface angles would have been just as easy to resolve, because we can draw the Hip rafter’s footprints. Ditto for height, the ridge lines need not intersect and the cross-sectional profiles could have been different sizes. As far as the upper surface angles are concerned, what seemed at first sight to be a mind-bending intersection is now a piece of cake.













Updated Drawings




Here's some 3D drawings for reference.








When Hips Collide – Version 2.0
Sim ... I don’t have much time so just here are a few images for the time being in case anyone wants to make a cardboard model as a visual reference for further study. The blade bevels are indicated on the development so a model can be cut from wood if desired (the cutting will likely be with a hand saw, I can't see doing this with a compound miter saw).
The mathematical analysis to produce the model was thorough: linear algebra and vectors, slope-intercept equations of lines, trigonometry, geometry (especially the all important DP lines and the planes that generate them), compound angle formulas. Overall I'm satisfied with the math and pleased with the model and hopefully all the bugs and faults have been worked out. If the fit seems a bit sloppy in the photos it's because the two pieces aren't taped or glued together, one is just balancing on the other.
Now comes the hard part – writing this up in a comprehensible format in terms of the developmental geometry. Let’s see if we can turn Mr. Hyde back into Dr. Jekyll ...
















Komentar

Posting Komentar

Postingan populer dari blog ini

Layover Valley Rafter Basics

Gambar
Before I get into the Hexenschnitt for the Layover Valley Rafter I needed to review the basic geometry that defines the layover valley rafter. I needed to make sure I could draw the layover valley rafter correctly in plan view (ground plan). Here's one method of a basic drawing using the layover valley rafter backing triangle to position the layover valley rafter material on the roof surface that defines the  layover valley rafter in plan view. The  layover valley rafter top bevel is easily defined by extending the  layover valley rafter backing triangle to the top of the  layover valley rafter. The other  layover valley rafter line in plan view is defined by the bottom edge of the  layover valley rafter material sitting on the  layover valley rafter backing triangle. (Roof Surface). The  layover valley rafter plan view lines at the intersection of the two roofs. I tried this method for drawing the  layover valley rafter, but this method...
Baca selengkapnya

Hip Rafter Edge Bevel and Backing Angle

Gambar
In complex roof framing there are 7 main roof framing angles. Hip Rafter Backing Angle Valley Sleeper Bevel Angle Plain Titl (DP) Main Plan Angle Adjacent Plan Angle  Main Profile Rafter Slope Angle Adjacent Profile Rafter Slope Angle In this article I will discuss the hip rafter backing angle and the valley sleeper bevel angle. These two angles determine the miter angle and and saw blade bevel angles for jack rafters and purlin rafters that are plumb to the earth and for jack rafters and purlin rafters that are skewed from the eave line. A thorough knowledge of these two angles is critical in complex roof framing using geometry or trigonometry. The hip rafter edge bevel was avoided in the 12th century on some roof structures by rotating the hip rafter into the roof surface plane. However, by avoiding the hip rafter edge bevel, the carpenters-builders had to have a knowledge of the Valley Sleeper Bevel Angle. They didn't call it the Valley Sleeper Bevel Angle, but it's the same...
Baca selengkapnya

Layover Valley Rafter Hexenschnitt #3

Gambar
In the book Das neue Buch vom alten Wissen der Schiftung The new book on the ancient knowledge Schiftung The new book on the ancient knowledge Roof Framing The new book on the ancient knowledge Roof Framing Geometry by Bernd Küppers I'm stuck on page 23. The geometry in the book, just doesn't return the correct miter angle for the Layover Valley Rafter Witches Cut (Hexenschnitt). I don't know if I'm drawing it incorrectly, or the BE (Bottom Edge Plane of the Layover Valley Rafter) should be drawn differently. Similar drawing at File: Kehlbohle mit Hexenschnitt.jpg There's 3 canted planes that define the Layover Valley Rafter Witches Cut Miter Angle. The main roof surface, the square tail fascia plane of the main roof and the the square tail fascia plane of the adjacent roof.  I think the problem with my geometric drawings is defining the intersection of the roof surface with the adjacent  square tail fascia plane.
Baca selengkapnya